Hello out there, ferris wheelers! I went through all your blog posts this week and pulled some snippets that really stood out to me, for many different reasons, but the one they all have in common besides general awesomeness is this:
They contain words that I never said.
The words, and therefore the thoughts, belong to you, they came from your brains, which means you stretched your brains beyond what happened during class, or a voicethread, or while you’re doing homework examples, or while you’re doing a test. That’s real learning, folks, it’s not about what I do or say, believe me.
And then you shared these thoughts with THE WORLD. Everybody wins!
Well done, all! Here are the snippets so far, as of this moment, which is Sunday lunchtime, but if more pop up between now and tomorrow, I’ll add them too:
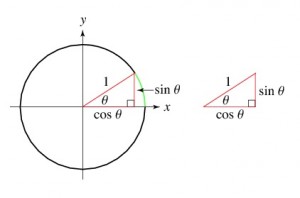
On the unit circle:
Lizzie, a truly neat formula for coterminal angles:
You can have different numbers for the angles by using a single “initial” angle. The most common angle would be : 90 degrees. So let’s say 90 degrees is your “initial angle”, it’s ‘synonyms’ can also be; 450 , 810 , 1170, even – 270 and -630 degrees. A mathematical rule for this example would be : 360 x + 90 = y where y= resulting degrees and x = full circle counter-clock .
Tyson, on finding a coterminal angle for a negative angle:
When an angle given to you is negative, don’t let it confuse you, the same process is used to find coterminal angles (adding 360).
On the Big 17:
Amanda, on how she remembers the radian angles by their denominators:
Basically, I separate the circle into sections; the “over” 1s, 2s, 3s, 4s and 6s. Then, in my mind I visualize the lines that would be there and place my values.
Jessica, using a visual relationship to remember the radian angles (and not the one we talked about in class, either):
I have worked on the ferris wheel with Javiera and we have both realized that there is a mirror image ( for the denominators) from left to right. also we have realized that the fractions that are the same color all around the circle, have the same denominator
Cassandra, another memory aid for radians:
My tip is that the first is a red cross which represents the quadrantal angles. Then we separate this cross into 4 pieces with yellow lines. Then we split the 180 degrees into 3 with pink lines. And finally we separate the 6 angles into halves with green lines.
Duncan, on remembering via UNDERSTANDING:
I think the easiest way to remember radians is simply to remember that 180 degrees is equal to pi radians. From there you can divey it up with fractions and the likes, 30 degrees equals pi/6, 90 degrees equals pi/2, 120 degrees equals 3pi/4, so on so forth.
David, a comment about commenting, which is the lifeblood of blogging, in my opinion:
(i saw and liked Amanda’s way that was the only post i saw so id love to know your tricks) or maybe you just have photographic memory which would be awesome.
David again, made me laugh in a comment on Marianne’s post:
My favorite part of your post is all of it and i also enjoy reading you other post you keep it orgainized short and simple which is wonderful….i also really like how you put the images big so we dont have to click on them we can see them within the blog post unlike mine ahaha
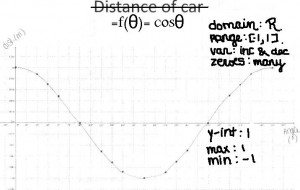
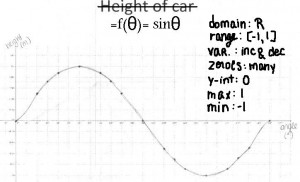
On the 2 basic functions:
Marianne: pictures that are worth 2pi-thousand words:
Justine, noticing something that will be SO important very soon:
Both graphs look like a wave ![]() Only the “height” one looks shifted to the left compared with the “distance from the wall” one.
Only the “height” one looks shifted to the left compared with the “distance from the wall” one.
Cassandra, also making me laugh, and I am so calling them this from now on:
Well today we learned the actual function to this cool wavy function that I call the “ferris wheel function” since I find “trig function” is boring. So we actually learned how to see SIN and COS in a different way.
Fred, with a great way to visually and conceptually link the ferris wheel to the functions:
…we have learned that if the wall is on the y axis and the ground on the x axis. both graphs will look exactly the same except that they won’t start at the same place.
Mason, with handy calculator tips:
Today in class we discovered that the rules for the 2 Ferris wheel graphs are y=cos(X) and y=sin(X) and how to graph it on your calculator; Which I will NEVER remember, but while I do, I’ll share it! LOL 1) Select Y= 2) Eneter in Y= sin (x) 3)press ZOOM 4) Choose Ztrig 5) Press Enter and Watch the line!
Tanyse, with a short but powerful summary of the big picture:
we worked on pulling information from our unit circles to form a table and then use that to graph……
Javiera, on what the basic functions have to do with the unit circle ferris wheel car:
So when we are making a graph for sin we are really graphing the distance from the floor according to time….when we are graphic cos we are really graphing the distance to the wall according to time
Javiera again, just an awesomely insightful and deep thought about why we made two graphs from the single ferris wheel (I seriously got goosebumps when I read this):
I think we do this because we have 3 variables going at the same time: The height, the distance to the wall and the time and since we only do 2 variables at the time we separate them into two different graphs and of course the time is the x since it is the independent variable
Elise, on the connection between trigonometry last year and this year:
I now understand where the trigonometry comes into account in the function.
David: on how to remember which function is which ( okay I did say this, but I like the emphasized spelling:
sIIIInn yIIIIII heIIIIIIght
coSSSS xSSSSS diSSSStanCCe
Tyson. the psychic student, thinking ahead to when we’re dealing with circles other than the unit circle, which, by the way, you actually already have:
To my knowledge, this method only works if the circle has a radius of 1 unit, so my guess is that if the circles radius is bigger than that, we will have to add that into the equation somehow.
On special properties of trig functions:
Cassandra, on how to recognize a cycle, and how to recognize a NON-cycle:
To remember: all cycle have the same width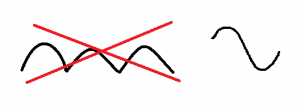
Eli, adding an important detail to my definition of frequency, which believe me will be there from now on:
The frequency-is equal to how many cycles there are up to 2pi= a full circle
Jack, using his own vocabulary which is all kinds of awesome:
…l.o.o or as i like to call it the equilibrium…..a cycle is a part of the curve that if you would put next to similar part it would make a wave. a period is the distance from one crest or trough to another….the amplitude is the distance between the crest or trough to the equilibrium.
Tyson, on frequency:
The way that I remember what the frequency is, is by looking at the word. Frequent means often, so frequency is how often something is happening within an x value of a graph
And my final words.…now that you have all done such amazing work, you do realize what this means, don’t you?
We are so going to keep doing this, folks. But tell me what you think, especially those of you who actually posted every day, do you think you understand this stuff better as a result? What about reading other peoples’ thoughts, either here or on their own blogs?